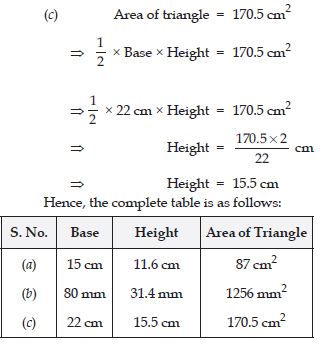
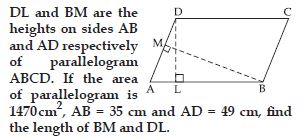
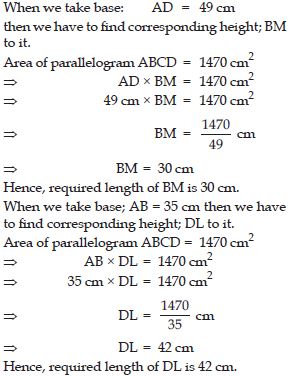
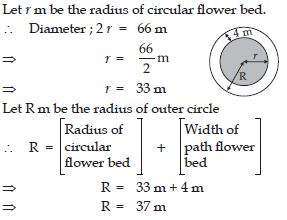
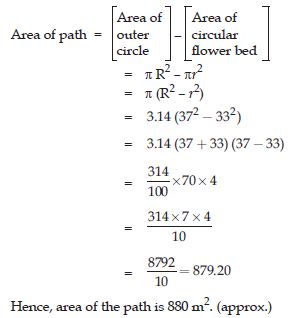
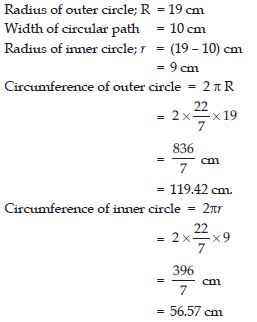
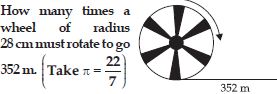
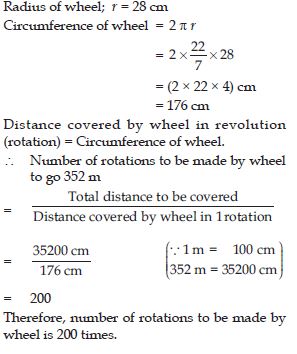
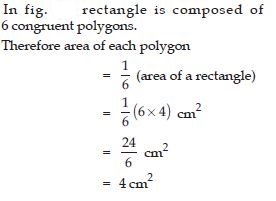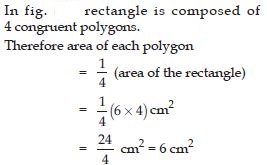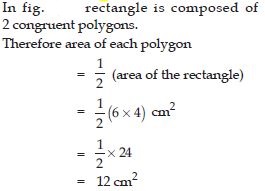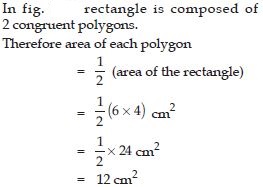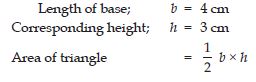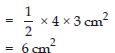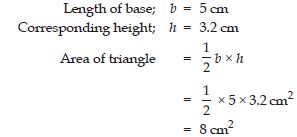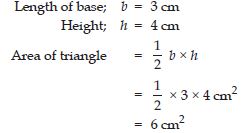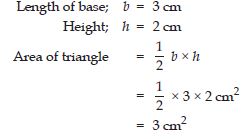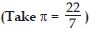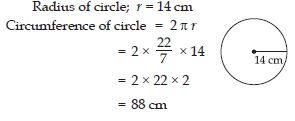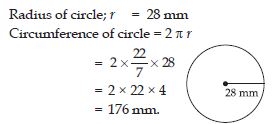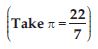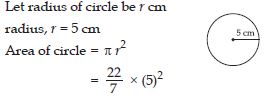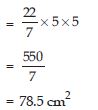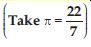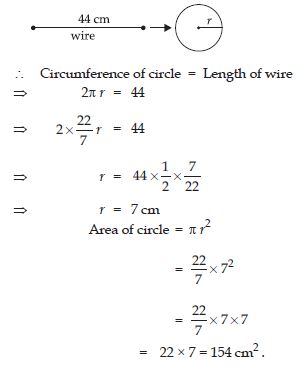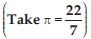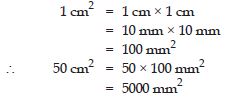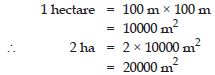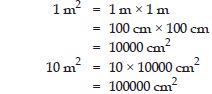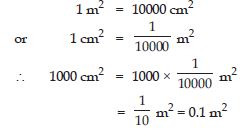Question 1:
Would you need to find, area or perimeter, to answer the following?
How much space does a blackboard occupy?
Answer:
We need to find area to determine the space occupied by a blackboard. Shaded region indicates the space occupied by a blackboard.
Question 2:
Would you need to find, area or perimeter, to answer the following?
What is the length of wire required to fence a rectangular flower bed?
Answer:
We need to find the perimeter of rectangular flower bed; for the wire required to fence a rectangular flower bed.
Question 3:
What distance would you cover by taking two rounds of a triangular park?
Answer:
Question 4:
How much plastic sheet do you need to cover a rectangular swimming pool?
Answer:
We find the area of rectangular swimming pool to cover it with plastic sheet.
Question 5:
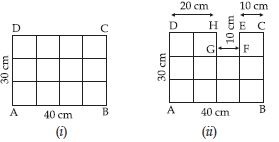
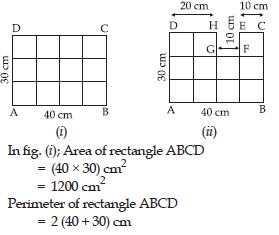
Compute their areas and perimeters.
Answer:
= 2 × 70 cm
= 140 cm
When we cut a square of side 10 cm from
rectangle ABCD, we get fig., (ii).
Area of remaining sheet = Area of rectangle
ABCD – Area of square EFGH
= (40 × 30) cm2 – (10 × 10) cm2
= (1200 – 100) cm2
= 1100 cm2
Perimeter of remaining sheet
= AB + BC + CE + EF + FG + GH + HD + AD
= (40 + 30 + 10 + 10 + 10 + 10 + 20 + 30) cm
= 160 cm
We observe that area of fig. (ii) decreases by
(1200 – 1100) cm2 i.e. by 100 cm2 but its perimeter
increases by (160 – 140) cm i.e. by 20 cm.
Question 6:
The length and the breadth of a rectangular piece of land is 500 m by 300 m respectively. Find its area.
Answer:
Question 7:
The length and the breadth of a rectangular piece of land is 500 m by 300 m respectively. Find the cost of the land, if 1 m2 of the land costs ₹10,000.
Answer:
Cost of 1 m2 land = ₹10000
Cost of 150000 m2 land = ₹(10000 × 150000)
= ₹1,50,00,00,000.
Question 8:
Find the area of a square park whose perimeter is 320 m.
Answer:
Question 9:
Find the breadth of a rectangular plot of land, if its area is 440 m2 and the length is 22 m. Also find its perimeter.
Answer:
Question 10:
The perimeter of a rectangular sheet is 100 cm. If the length is 35 cm, find its breadth. Also find the area.
Answer:
Question 11:
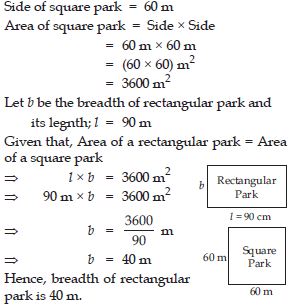
The area of a square park is the same as of a rectangular park. If the side of the square park is 60 m and the length of the rectangular park is 90 m, find the breadth of the rectangular park.
Answer:
Question 12:
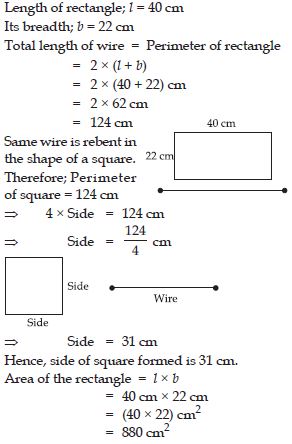
A wire is in the shape of a rectangle. Its length is 40 cm and breadth is 22 cm. If the same wire is rebent in the shape of a square, what will be the measure of each side? Also find which shape encloses more area?
Answer:
Area of the square = (Side)2
= 31 cm × 31 cm
= (31 × 31) cm2
= 961 cm2
So, the square encloses more area even though
its perimeter is same as that of the rectangle.
Question 13:
The perimeter of a rectangle is 130 cm. If the breadth of the rectangle is 30 cm, find its length. Also find the area of the rectangle.
Answer:
Question 14:
Answer:
Question 15:
Each of the following rectangles of the length 6 cm and the breadth 4 cm is composed of congruent polygons. Find the area of each polygon.
Answer:
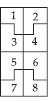
Question 16:
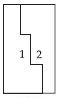
Each of the following rectangles of the length 6 cm and the breadth 4 cm is composed of congruent polygons. Find the area of each polygon.
Answer:
Question 17:
Each of the following rectangles of the length 6 cm and the breadth 4 cm is composed of congruent polygons. Find the area of each polygon.
Answer:
Question 18:
Each of the following rectangles of the length 6 cm and the breadth 4 cm is composed of congruent polygons. Find the area of each polygon.
Answer:
Question 19:
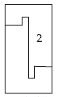
Each of the following rectangles of the length 6 cm and the breadth 4 cm is composed of congruent polygons. Find the area of each polygon.
Answer:
Question 20:
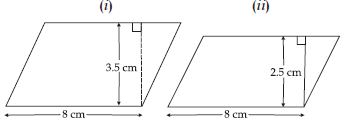
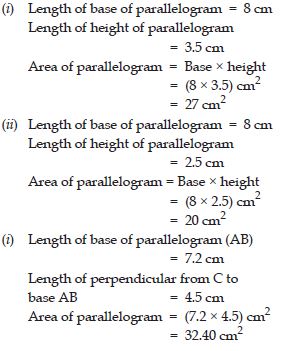
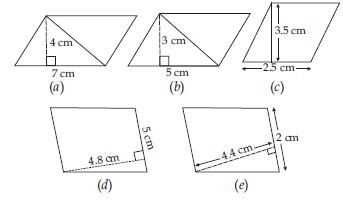
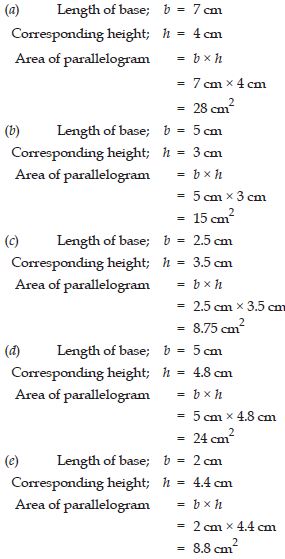
Find the area of following parallelograms:
(iii) In parallelogram ABCD, AB = 7.2 cm and the perpendicular from C on AB is 4.5 cm.
Answer:
Question 21:
Find the area of each of the following parallelograms:
Answer:
Question 22:
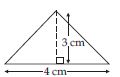
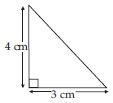
Find the area of the following triangles:
Answer:
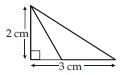
Question 23:
Find the area of the following triangles:
Answer:
Question 24:
Find the area of the following triangles:
Answer:
Question 25:
Find the area of the following triangles:
Answer:
Question 26:
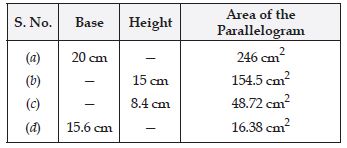
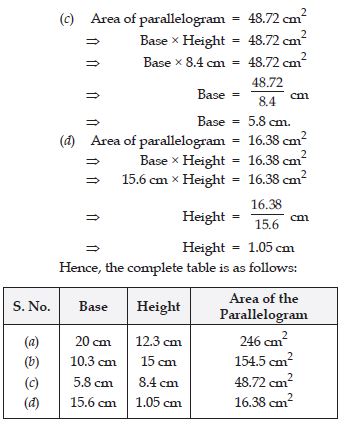
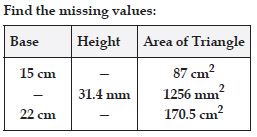
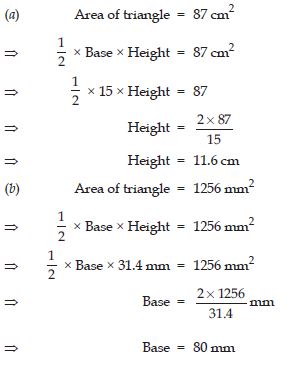
Find the missing values:
Answer:
Question 27:
Answer:
Question 28:
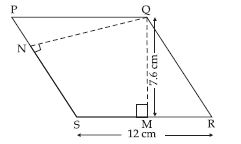
PQRS is a parallelogram. QM is the height from Q to SR and QN is the height from Q to PS. If SR = 12 cm and QM = 7.6 cm, then find the area of the parallelogram PQRS
Answer:
Question 29:
PQRS is a parallelogram. QM is the height from Q to SR and QN is the height from Q to PS. If SR = 12 cm and QM = 7.6 cm, then find QN, if PS = 8 cm.
Answer:
Question 30:
Answer:
Question 31:
Answer:
Question 32:
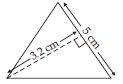
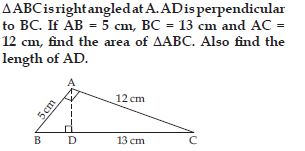
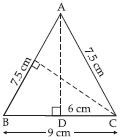
ABC is isosceles with AB = AC = 7.5 cm and BC = 9 cm. The height AD from A to BC, is 6 cm. Find the area of ABC. What will be the height from C to AB i.e., CE?
Answer:
Base; BC = 9 cm
Corresponding height; AD = 6 cm
Question 33:
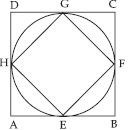
Which square has the larger perimeter?
Answer:
Outer square ABCD has the larger perimeter.
Justification:
Let side of square ABCD be 200 cm.
On measuring each side of square EFGH.
We find that
EF = FG = GH = EH = 141 cm
Now perimeter of square ABCD = (4 × 200) cm
= 800 cm
Perimeter of square EFGH = (4 × 141) cm
= 564 cm
Hence, perimeter of outer square ABCD is larger.
Question 34:
Which is larger, perimeter of smaller square or circumference of the circle?
Answer:
On measuring diagonal EG of square EFGH; we
find that
EG = 199 cm approximately.
Diameter of circle = EG = 199 cm
Circumference of circle = d
= (3.14 × 199) cm
= 625 cm approximately
Perimeter of smaller square
EFGH = (4 × 141) cm
= 564 cm
We observe that circumference of circle is larger
than the perimeter of smaller square EFGH.
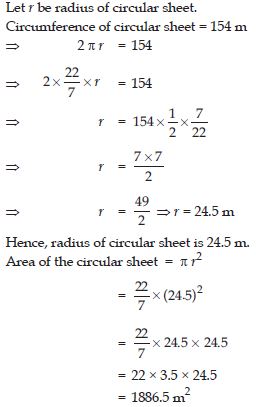
Question 35:
Find the circumference of the circles with the following radius:
Answer:
Question 36:
Find the circumference of the circles with the following radius:
28 mm
Answer:
Question 37:
Find the circumference of the circles with the following radius:
21 cm.
Answer:
Question 38:
Find the area of the following circles, given that
radius = 14 mm
Answer:
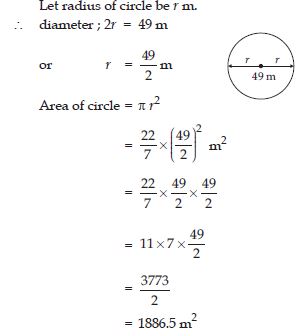
Question 39:
Find the area of the following circles, given that
Answer:
Question 40:
Find the area of the following circles, given that
radius = 5 cm
Answer:
Question 41:
Answer:
Question 42:
Answer:
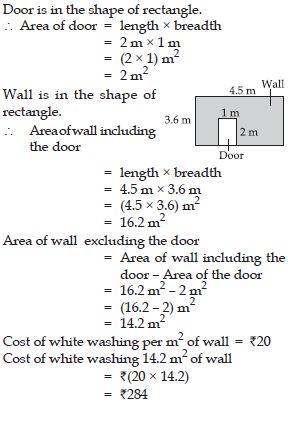
Now cost of 1 m rope = ₹4
Cost of 132 m rope = ₹(4 × 132)
= ₹528
Question 43:
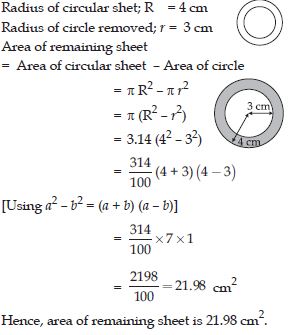
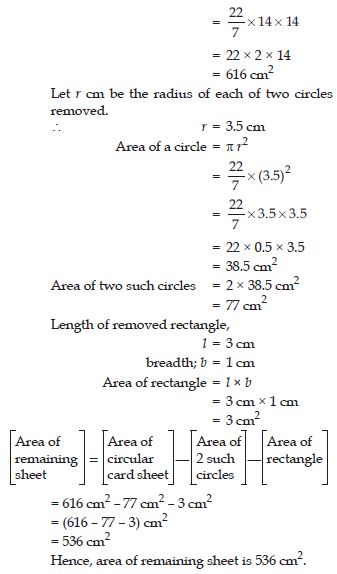
From a circular of radius 4 cm, a circle of radius 3 cm is removed (fig). Find the area of the remaining sheet. (Take = 3.14)
Answer:
Question 44:
Saima wants to put a lace on the edge of a circular table cover of diameter 1.5 m. Find the length of the lace required and also find its cost if one metre of the lace costs ₹15. (Take = 3.14)
Answer:
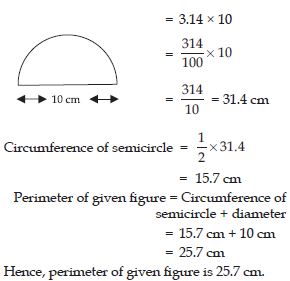
Question 45:
Find the perimeter of following figure, which is a semicircle including its diameter.
Answer:
Diameter ; d = 10 cm
Circumference of circle = d
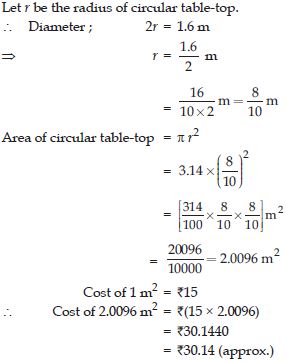
Question 46:
Find the cost of polishing a circular table-top of diameter 1.6 m, if the rate of polishing is ₹15 m2. (Take = 3.14)
Answer:
Question 47:
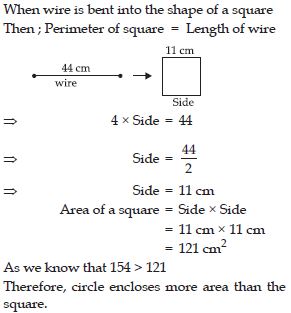
Shazli took a wire of length 44 cm and bent it into the shape of a circle. Find the radius of that circle. Also find the area. If the same wire is bent into the shape of a square, what will be the length of each of its sides? Which figure encloses more area, the circle or square?
Answer:
Let r cm be the radius of circle.
Wire of length 44 cm is bent into the shape of a
circle.
Question 48:
Answer:
Question 49:
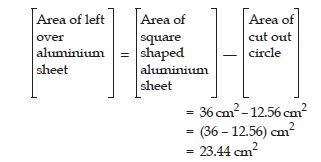
A circle of radius 2 cm is cut out from a square piece of an aluminium sheet of side 6 cm. What is the area of the left over aluminium sheet? (Take = 3.14)
Answer:
Question 50:
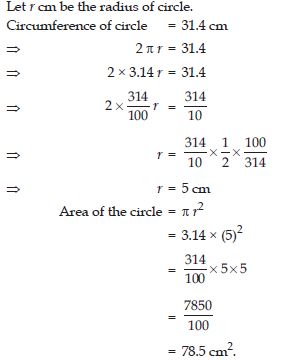
The circumference of a circle is 31.4 cm. Find the radius and the area of the circle? (Take = 3.14)
Answer:
Question 51:
Answer:
Question 52:
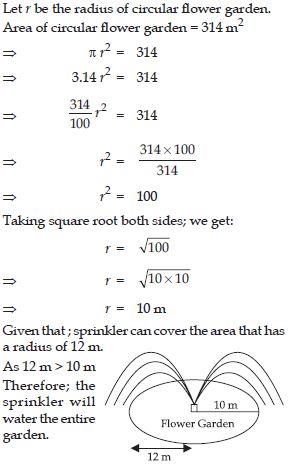
A circular flower garden has an area of 314 m2. A sprinkler at the center of the garden can cover an area that has a radius of 12 m. Will the sprinkler water the entire garden? (Take = 3.14)
Answer:
Question 53:
Answer:
Question 54:
Answer:
Question 55:
The minute hand of a circular clock is 15 cm long. How far does the tip of the minute hand move in 1 hour? (Take = 3.14)
Answer:
Question 56:
50 cm2 in mm2
Answer:
Question 57:
2 ha in m2
Answer:
Question 58:
10 m2 in cm2
Answer:
Question 59:
1000 cm2 in m2
Answer:
Question 60:
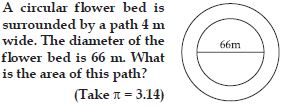
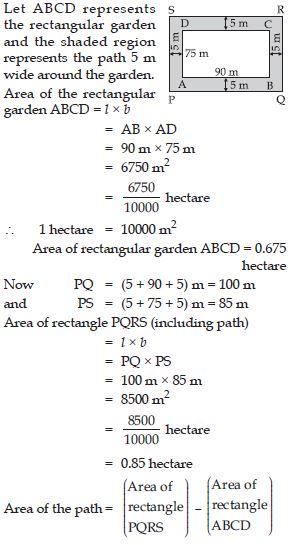
A garden is 90 m long and 75 m broad. A path 5 m wide is to be built outside around it. Find the area of the path. Also find the area of the garden in hectare.
Answer:
= (0.85 – 0.675) hectare
= 0.175 hectare
Hence, area of the path is 0.175 hectare and area
of the garden is 0.675 hectare
Question 61:
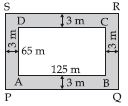
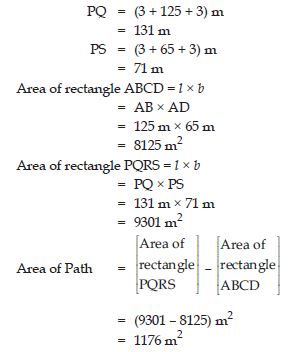
A 3 m wide path runs outside around a rectangular park of the length 125 m and the breadth 65 m. Find the area of the path.
Answer:
Let ABCD represents the rectangular park and
the shaded region represents the path 3 m wide
around the park.
To know the area of the path we have to subtract
the area of rectangle ABCD from the area of
the rectangle PQRS.
Question 62:
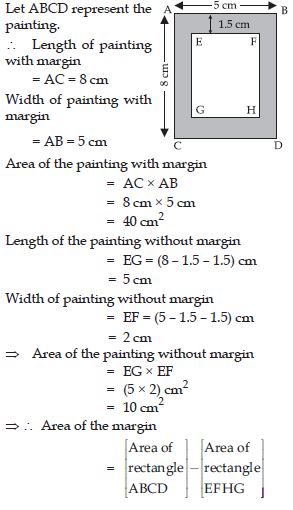
A picture is painted on a cardboard 8 cm long and 5 cm wide such that there is a margin of 1.5 cm along each of its sides. Find the total area of the margin.
Answer:
= 40 cm2 – 10 cm2
= 30 cm2
Question 63:
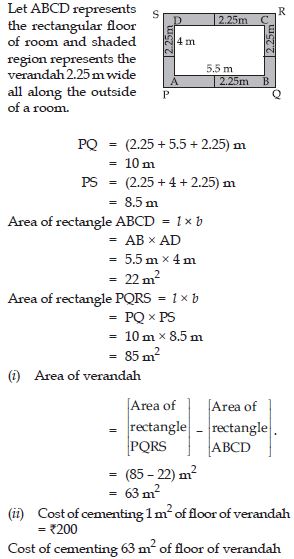
A verandah of width 2.25 m wide is constructed all along outside of a room which is 5.5 m
long and 4 m wide. Find:
(i) the area of the verandah.
(ii) the cost of cementing the floor of the verandah at the rate of ₹200 per m2.
Answer:
= ₹(200 × 63)
= ₹12,600.
Question 64:
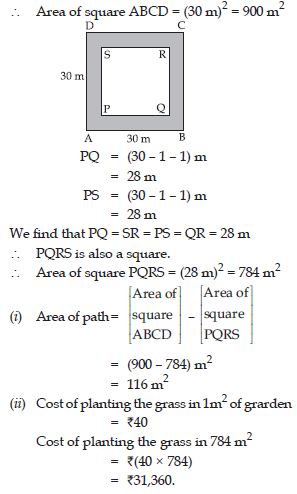
A path 1 m wide is built along the border inside a square garden of side 30 m. Find:
(i) the area of the path
(ii) the cost of planting the grass in the remaining portion of the garden at the rate of
₹40 per m2.
Answer:
Let ABCD represent the square garden of side
30 m and shaded region represents the path
1 m wide; built along inside the square garden.
Question 65:
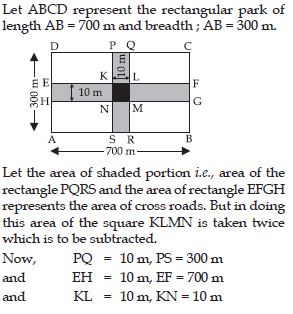
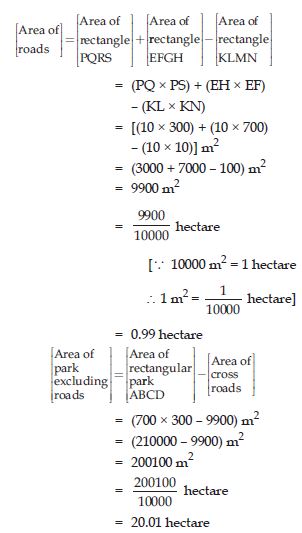
Two cross roads, each of width 10 m, cut at right angles through the centre of a rectangular park of the length 700 m and the breadth 300 m parallel to its sides. Find the area of the roads. Also find the area of park excluding cross roads give the answer in hectarees.
Answer:
Question 66:
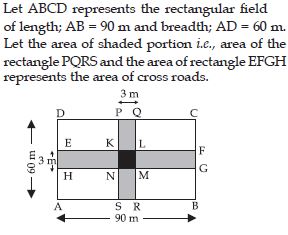
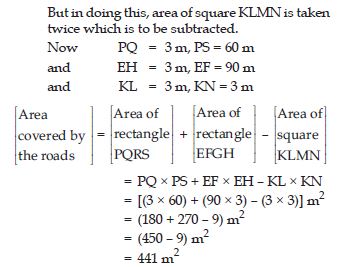
Through a rectangular field of the length 90 m and the breadth 60 m, two roads are
constructed which are parallel to the sides and cut each other at right angles through the
centre of the fields. If the width of each road is 3 m, find
(i) the area covered by the roads.
(ii) the cost of constructing the roads at the
rate of ₹110 per m2.
Answer:
(i)
(ii) Cost of constructing 1 m2 of roads = ₹110
Therefore, cost of constructing 441 m2 of roads
= ₹(110 × 441)
= ₹48510.
Question 67:
Pragya wrapped a cord around a circular pipe of radius 4 cm (shown below) and cut off the length required of the cord. Then she wrapped it around the square box of the side 4 cm (also shown). Did she have any cord left? ( = 3.14)
Answer:
Circumference of circle = 2r
= 2 × 3.14 × 4
= 25.12 cm
Perimeter of square box of side 4 cm
= 4 side
= (4 × 4) = 16 cm
We find that when cord of length 25.12 cm is
used to wrap the square box of side 4 cm only
16 cm of cord is used. Therefore she was left
with (25.12 – 16) cm i.e., 9.12 cm chord.
Question 68:
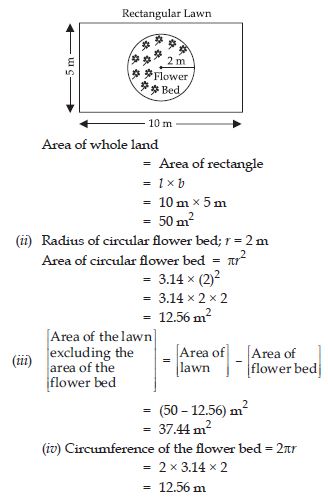
The figure represents a rectangular lawn with a circular flower bed in the middle. Find:
(i) the area of whole land
(ii) the area of flower bed
(iii) the area of the lawn excluding the area of the flower bed.
(iv) the circumference of the flower bed.
Answer:
(i) Length of rectangular lawn; l = 10 m
Its breadth; b = 5 m
Question 69:
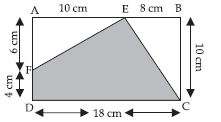
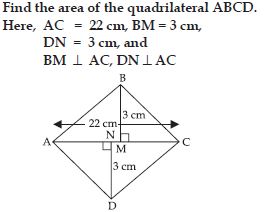
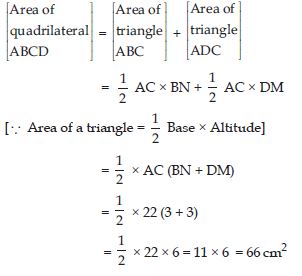
In the following figures, find the area of shaded portion.
Answer:
Area of shaded portion EFDC
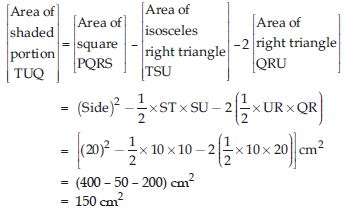
Question 70:
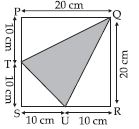
Find the area of shaded portion.
Answer:
In fig., all sides of quadrilateral PQRS are at right
angles and SR = 20 cm and QR = 20 cm.
Hence, PQRS is a square.
Now;