Question 1:
Which one of the following methods of scale is a universal method?
- Simple Statement
- Representative Fraction
- Graphical Scale
- None of the above
Answer:
(b). Representative Fraction
Question 2:
Map distance in a scale is also known as :
- Numerator
- Denominator
- Statement of Scale
- Representative Fraction
Answer:
(a). Numerator
Question 3:
‘Numerator’ in scale represents:
- Ground distance
- Map distance
- Both the distance
- None of the above
Answer:
(b). Map distance
Question 4:
What are the two different systems of measurement?
Answer:
Metric and British.
Question 5:
Give one example each of statement of scale in Metric and English system.
Answer:
1 metre : 100 cms, 1'' : 6 inch.
Question 6:
Why is the Representative Fraction method called a Universal method?
Answer:
It can be used in any measurement system.
Question 7:
What are the major advantages of the graphical method?
Answer:
It can be used is measure distance on a scale.
Question 8:
Convert the given statement of scale into representative fraction (RF) : 5 cm represents 10 km.
Answer:
1 kilometre = 1,00,000 cm.
∴ 10 km = 10,00,000 cm
So, 5 cm = 10,00,000 cm (10 km = 10,00,000 cm)
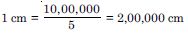
So, 1 cm = 2,00,000 cm
R.f = 1:2,00,000
Question 9:
Convert the given statement of scale into representative fraction (RF) : 2 inch represents 1 mile
Answer:
1 mile = 63,360 inch
∴ 4 mile = 63,360 × 4 = 2,53,440 inch
So, 2 inch = 2,53,440 inch
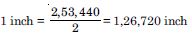
Ref. = 1:1,26,720
Question 10:
Convert the given statement of scale into representative fraction (RF) : 1 inch represents 1 yard
Answer:
1 yard = 36 inch R.F = 1:36
Question 11:
Convert the given statement of scale into representative fraction (RF) : 1 cm represents 100 metres
Answer:
1 cm = 10,000 cm
∴ 1 metre = 10,000 cm
R : F = 1:10,000
Question 12:
Convert the given R.F into the statement of scale in the system of measurements shown in parenthese : 1:100,000 (into km)
Answer:
1 cm, represents 1 km. Because, 1 km = 1,00,000 cm
Question 13:
Convert the given R.F into the statement of scale in the system of measurements shown in parenthese : 1:31,680 (into furlongs)
Answer:
1 cm, represents 1 km. Because, 1 km = 1,00,000 cm
Question 14:
Convert the given R.F into the statement of scale in the system of measurements shown in parenthese : 1:31,680 (into furlongs)
Answer:
1 inch represents 4 furlongs Because, 1 furlong–7, 920 inch So 31,680 inch = 4 furlong.
Question 15:
Convert the given R.F into the statement of scale in the system of measurements shown in parenthese : 1:126,720 (into miles)
Answer:
1 inch represents 2 mile Because, 1 mile = 63,360 inch So, 1,26,726 inch = 2 mile
Question 16:
Convert the given R.F into the statement of scale in the system of measurements shown in parenthese : 1:50,000 (into metres).
Answer:
1 cm represents 500 metre Because 1 mitre = 100 cm So, 50,000 cm = 500 metre Because = 1 km = 1,00,000 cm.
Question 17:
Construct a graphical scale when the given R.F is 1:50,000 and react the distances in kilometre and metre.
Answer:
Following steps may be followed to get the
length of line for a graphical scale.
Meaning of 1:50,000 is 1 unit of the may represents
50,000 ground units means 1 cm represents 50,000 cm.
or 15 cm = 50,000 × 15/100,000 km. or 15 cm = 7.5 km.
Because 7.5 km is not a round number, so we can
choose 5 or 10 (km) as a round number. In this question
we choose 5 as the round number.
For the length determination of the line to show
5 km following calculations to be carried our.
7.5 km represented by a line of 15 cm
5 km represented by a line of 15 × 5/7.5
Construction some steps are:
1. Firestly grow a line of 10 cm (straight line) and
divide this into sequal parts and assign a value of 1 km
each for 4 right side divisions from O mark.
2. Now divide the left side division as 10 equal parts
and mark every division by a value of 100 metres, start
from O.

when the given statement of scale is 1 inch
representing 1 mile construct a graphical scale & read
the distances in miles or furlongs.
