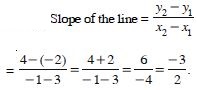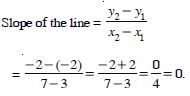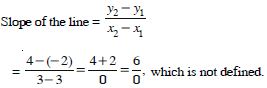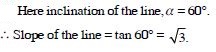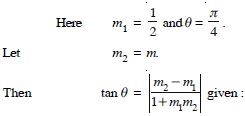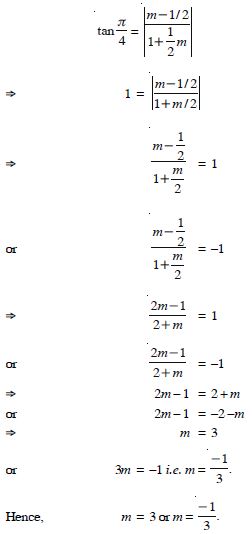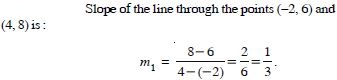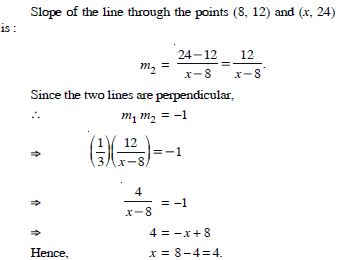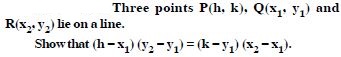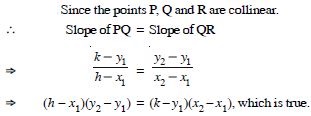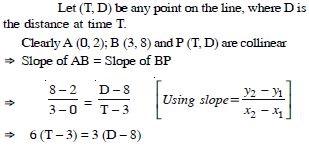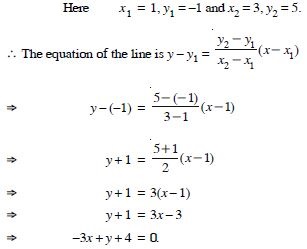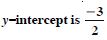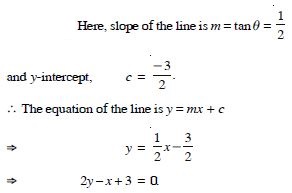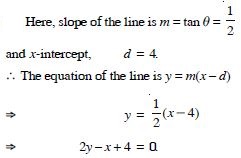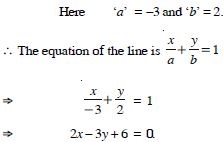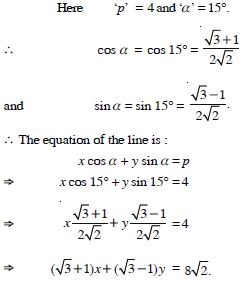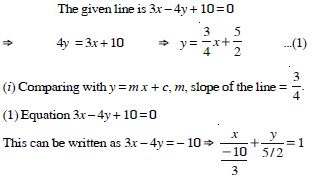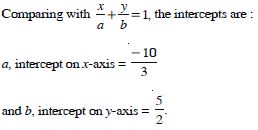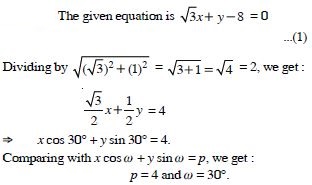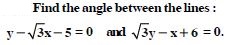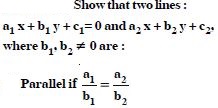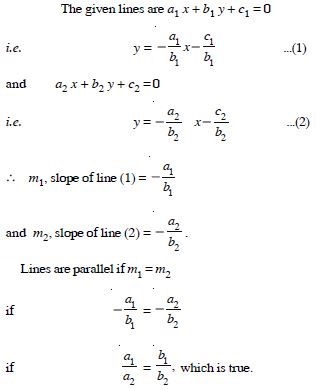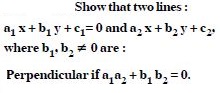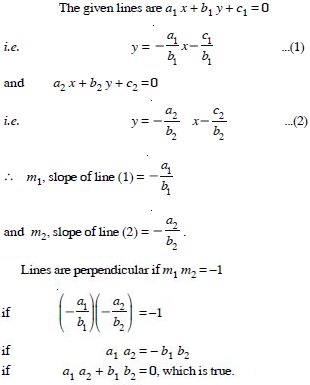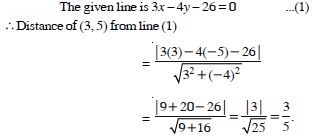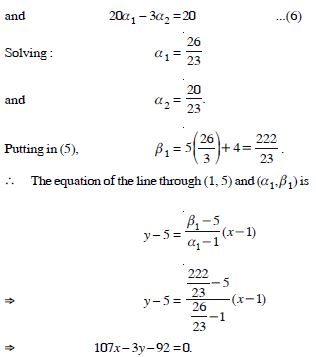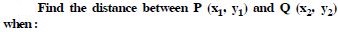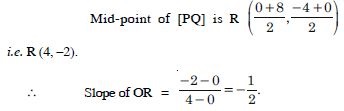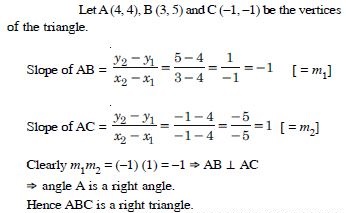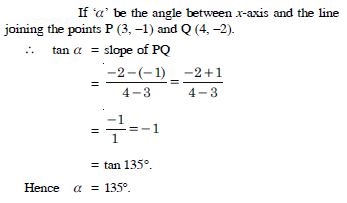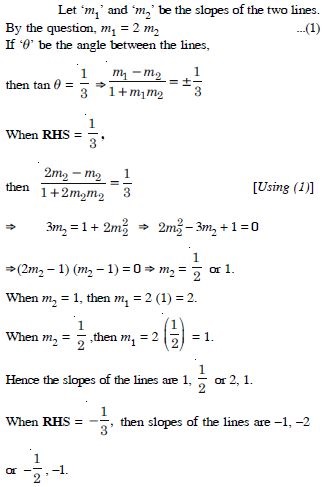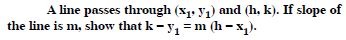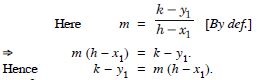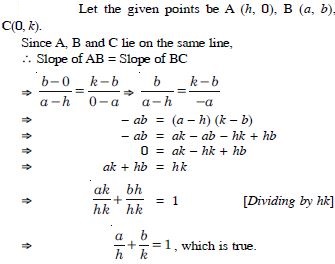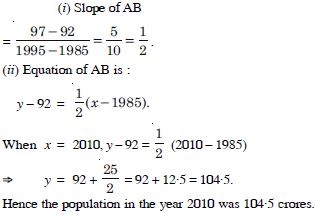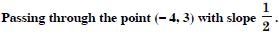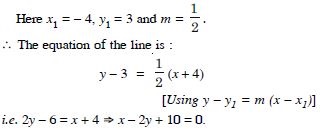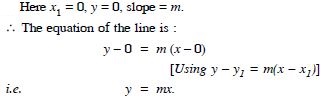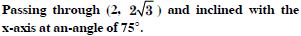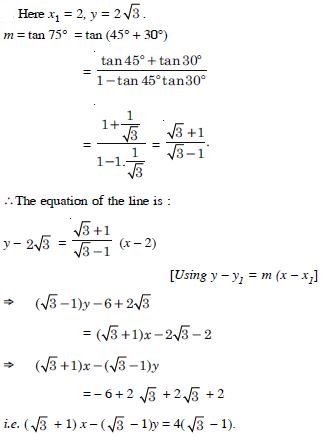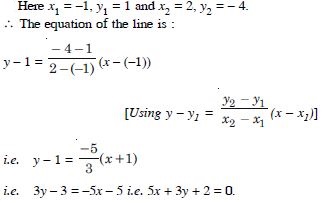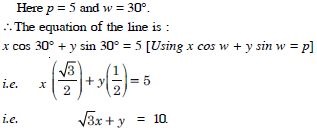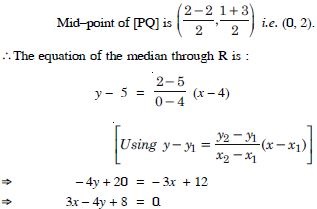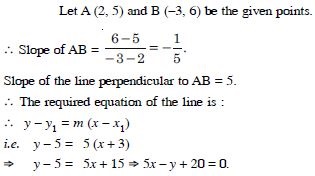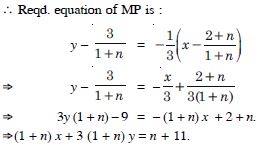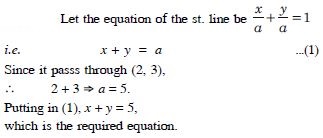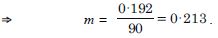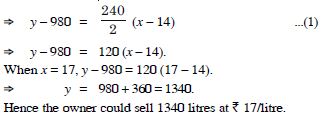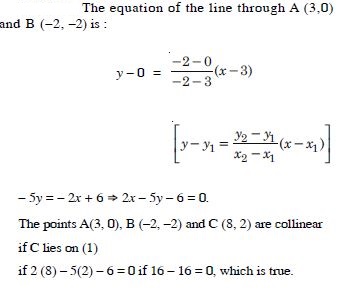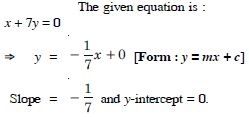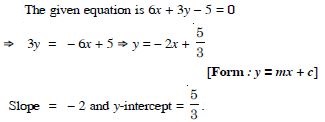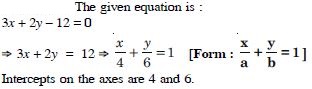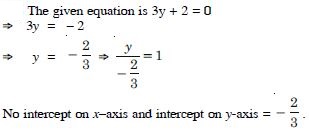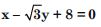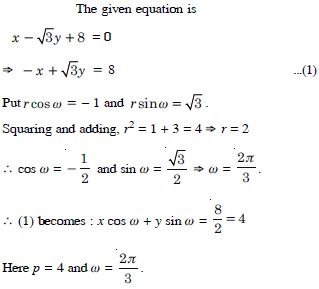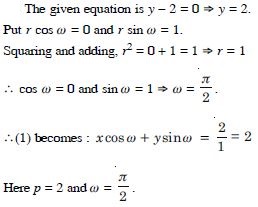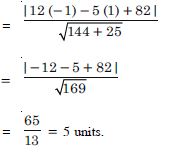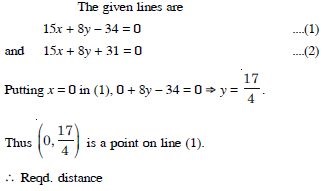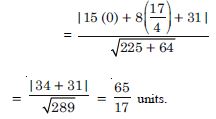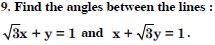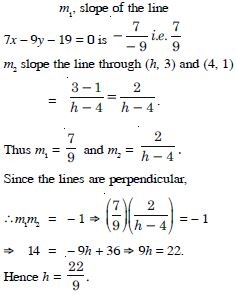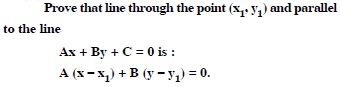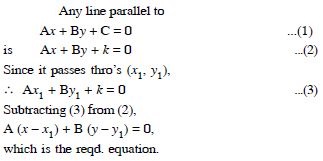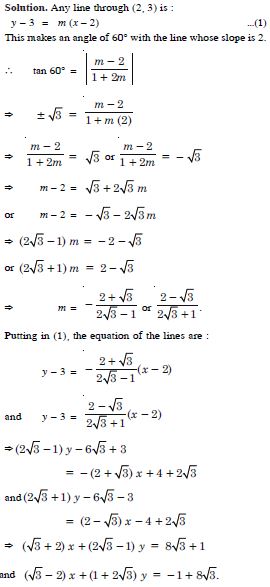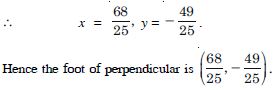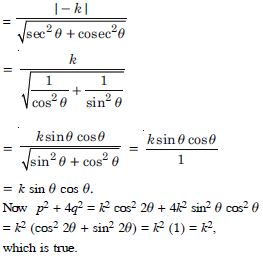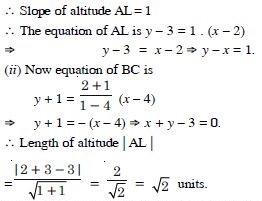Enhance your performance for the exams with free NCERT Solutions at Aasoka. These are very helpful even for competitive exam preparations such as JEE and NEET. The NCERT Solutions for Class 11 covers the entire chapter properly so that students do not miss out on anything important. The experts, at Aasoka, have made sure that students are able to master the concepts in no time. So, get on with the exam preparations now.
The “Straight Lines” chapter of Maths Class 11 teaches general equation of a line, slope of a line, the distance of a point from a line, various forms of equations of a line: slope-intercept form, normal form, parallel to axes, intercepts form, point-slope form, two-point form; the angle between two lines, and much more.
Question 1:
Find the slope of the line :
Passing through the points (3, –2) and (–1
Answer:
Question 2:
Find the slope of the line :
Passing through the points (3, –2) and (7, –2)
Answer:
Question 3:
Find the slope of the line :
Passing through the points (3, –2) and (3, 4)
Answer:
Question 4:
Find the slope of the line :
Making inclination of 60 with the positive direction
of x-axis.
Answer:
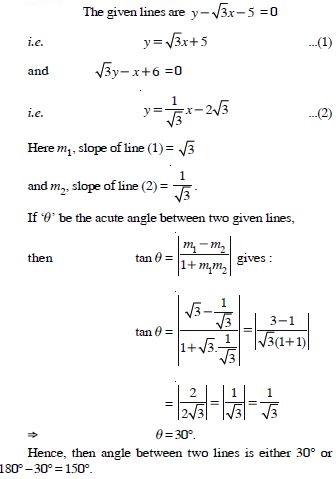
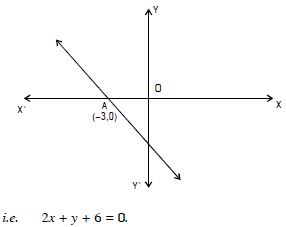
Question 5:
Answer:
Question 6:
Line through the points (–2, 6) and (4, 8) is perpendicular to the line through the points (8, 12) and (x, 24). Find the value of ‘x’.
Answer:
Question 7:
Answer:
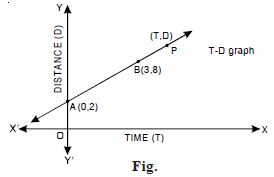
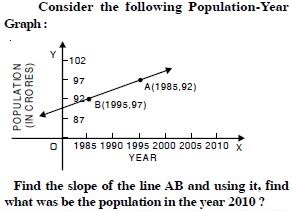
Question 8:
In the figure, time-distance graph of a
linear relation is given :
Two positions of time and distance recorded as :
When T = 0, D = 2 and when T = 3, D = 8.
Using the concept of slope, find the law of motion
i.e. how distance depends upon time.
Answer:
2 (T – 3) = D – 8 D = 2 (T + 1), which is the reqd. relation.
Question 9:
Find the equations of the lines parallel to axes and passing through (–2, 3).
Answer:
(i) Any line parallel to x-axis is y = b. ...(1)
Since it passes through (–2, 3),
3 = b
b = 3.
Putting in (1), y = 3, which is the required equation.
(ii) Any line parallel to y-axis is x = a ...(1)
Since it passes through (–2, 3),
–2 = a
a = –2.
Putting in (1), x = –2, which is the required equation.
Question 10:
Find the equation of line through (–2, 3) with slope – 4.
Answer:
The equation of the line is y – y1 = m(x – x1)
y – 3 = – 4[x – (–2)]
y – 3 = – 4(x + 2)
y – 3 = – 4x – 8
4x + y + 5 = 0.
Question 11:
Write the equation of the line through the points (1, –1) and (3, 5).
Answer:
Question 12:
Answer:
Question 13:
x-intercept is 4
Answer:
Question 14:
Find the equation of the line, which makes intercepts –3 and 2 on the x- and y-axes respectively.
Answer:
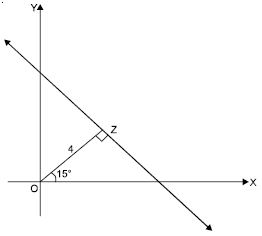
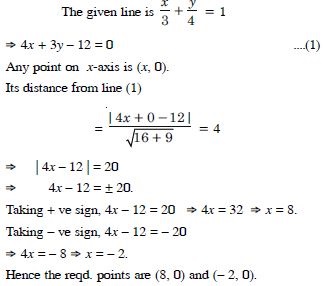
Question 15:
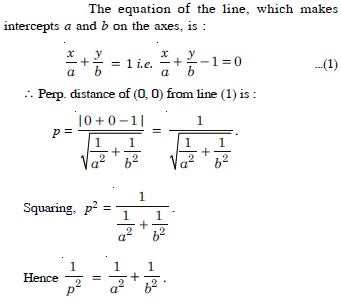
Find the equation of the line whose perpendicular distance from the origin is 4 units and the angle which the normal makes with positive direction of x-axis is 15.
Answer:
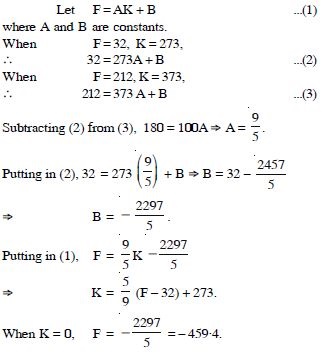
Question 16:
The Fahrenheit temperature F and absolute temperature K satisfy a linear equation. Given K = 273 when F = 32 and that K = 373 when F = 212. Express K in terms of F and the value of F when K = 0.
Answer:
Question 17:
Equation of a line is 3x – 4y + 10 = 0. Find its :
(i) slope (ii) x- and y-intercepts
Answer:
Question 18:
Answer:
Question 19:
Answer:
Question 20:
Answer:
Question 21:
Answer:
Question 22:
Find the equation of a line perpendicular to the line x – 2y + 3 = 0 and passing through the point (1, –2).
Answer:
Question 23:
Find the distance of the point (3, –5) from the line 3x – 4y – 26 = 0.
Answer:
Question 24:
Find the distance between the parallel
lines :
3x – 4y + 5 = 0 and 3x – 4y + 7 = 0.
Answer:
Question 25:
If the lines :
2x + y – 3 = 0, 5x + ky – 3 = 0 and 3x – y – 2 = 0
are concurrent, find the value of ‘k’.
Answer:
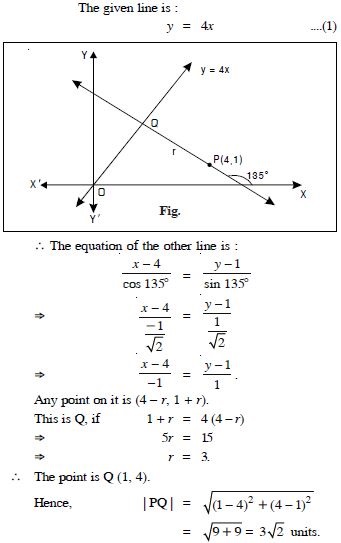
Question 26:
Find the distance of the line 4x – y = 0 from the point P(4, 1) measured along the line making an angle of 135 with the positive x-axis.
Answer:
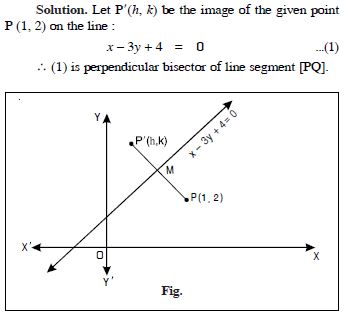
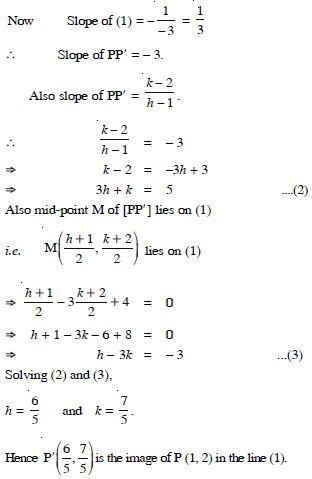
Question 27:
Assuming that straight line works as the plane mirror for a point, find the image of the point (1, 2) in the line x – 3y + 4 = 0.
Answer:
Question 28:
Answer:
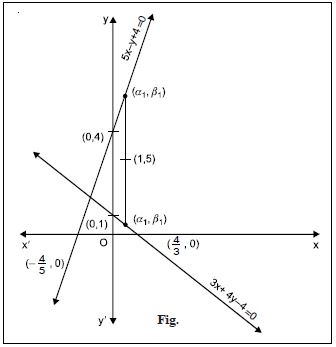
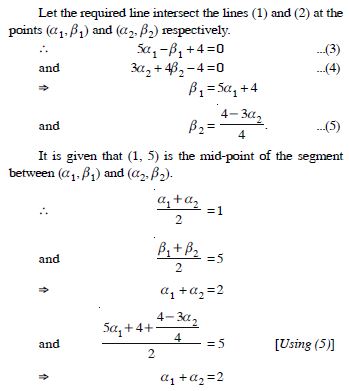
Question 29:
A line is such that its segment between the
lines :
5x – y + 4 = 0 and 3x + 4y – 4 = 0
is bisected at the point (1, 5). Obtain its equation.
Answer:
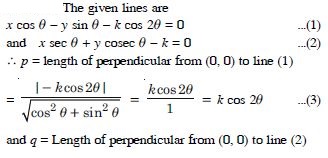
The given lines are ;
5x – y + 4 = 0 ...(1)
and 3x + 4y – 4 = 0 ...(2)
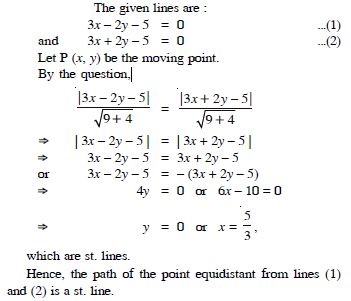
Question 30:
Show that the path of a moving point such
that its distance from the lines :
3x – 2y = 5 and 3x + 2y = 5
are equal, is a straight line.
Answer:
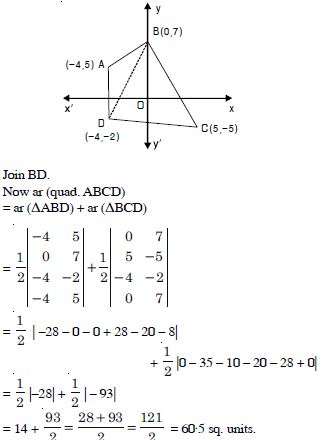
Question 31:
Draw a quadrilateral in the cartesian plane whose vertices are (– 4, 5), (0, 7), (5, –5) and (– 4, –2). Also find its area.
Answer:
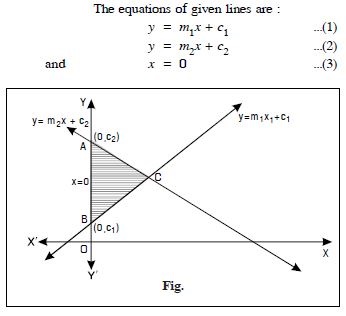
Let A (– 4, 5), B (0, 7), C (5, –5) and D (–4, –2) be the vertices of the quad. ABCD, which is as shown in the figure.
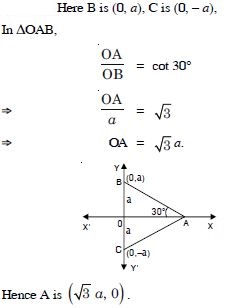
Question 32:
The base of an equilateral triangle with side 2a lies along the y-axis such that the mid–point of the base is the origin. Find the vertices of the triangle.
Answer:
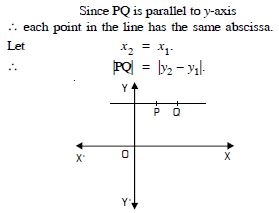
Question 33:
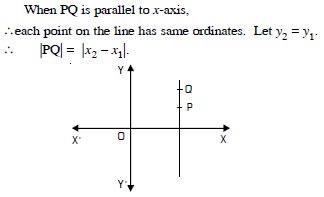
PQ is parallel to y-axis
Answer:
Question 34:
PQ is parallel to x-axis
Answer:
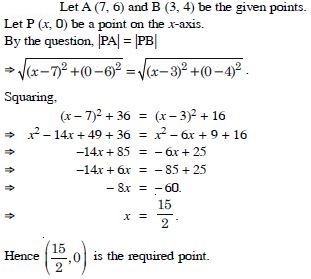
Question 35:
Find a point on the x-axis, which is equidistant from (7, 6) and (3, 4).
Answer:
Question 36:
Find the slope of the line, which passes through the origin and the mid-point of the line segment joining the points P (0, –4) and Q (8, 0).
Answer:
Question 37:
Without using Pythagorean Theorem, show that (4, 4), (3, 5) and (–1, –1) are vertices of a right triangle.
Answer:
Question 38:
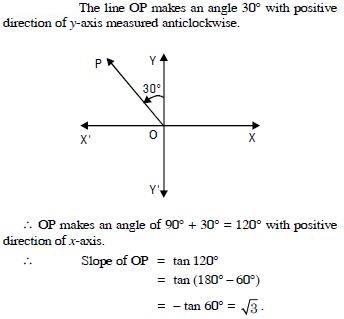
Find the slope of the line which makes an angle of 30 with the positive direction of y-axis, measured anticlockwise.
Answer:
Question 39:
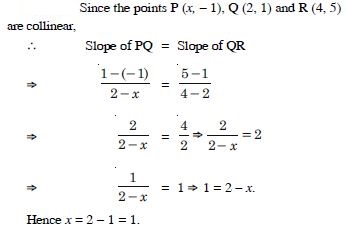
Find the value of x for which the points (x, –1), (2, 1) and (4, 5) are collinear.
Answer:
Question 40:
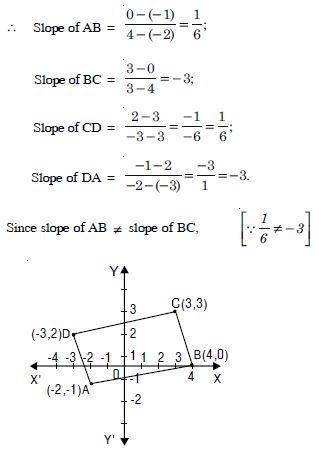
Without using the distance formula, show that (–2, –1), (4, 0), (3, 3) and (–3, 2) are the vertices of a parallelogram.
Answer:
Let A (–2, –1), B (4, 0), C (3, 3) and D (–3, 2) be the given points.
points A, B and C are not collinear.
Similarly, the points A, D and C are not collinear.
Thus the given points form a quadrilateral.
Now slope of AB = slope of CD
AB || CD
and slope of BC = slope of DA BC || DA.
Hence the given points form a parallelogram.
Question 41:
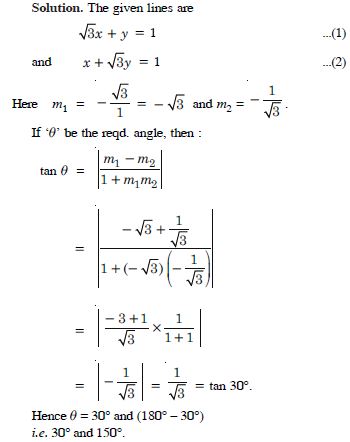
Find the angle between the x-axis and the line joining the points (3, –1) and (4, –2).
Answer:
Question 42:
Answer:
Question 43:
Answer:
Question 44:
Answer:
Question 45:
Answer:
Question 46:
find the equation of the line, which satisfy the given conditions :
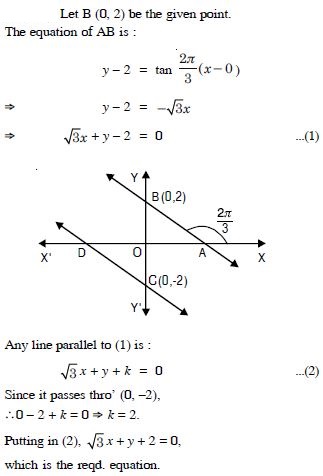
Write the equations for the x- and y-axis.Answer:
Equation of the x-axis is y = 0.
Equation of the y-axis is x = 0
Question 47:
find the equation of the line, which satisfy the given conditions :
Answer:
Question 48:
find the equation of the line, which satisfy the given conditions :
Passing through (0, 0) with slope m.Answer:
Question 49:
find the equation of the line, which satisfy the given conditions :
Answer:
Question 50:
find the equation of the line, which satisfy the given conditions :
Intersecting the x-axis at a distance of 3 units to the left of origin with slope -2.Answer:
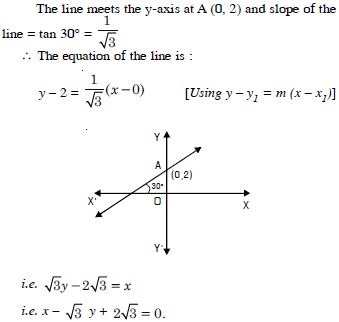
Question 51:
find the equation of the line, which satisfy the
given conditions :
Intersecting the y-axis at a distance of 2 units above
the origin and making an angle of 30 with positive
direction of the x-axis.
Answer:
Question 52:
find the equation of the line, which satisfy the
given conditions :
Passing through the points (–1, 1) and (2, –4).
Answer:
Question 53:
find the equation of the line, which satisfy the
given conditions :
Perpendicular distance from the origin is 5 units and
the angle made by the perpendicular with the positive
x-axis is 30.
Answer:
Question 54:
The vertices of a triangle PQR are P (2, 1), Q (–2, 3) and R (4, 5). Find the equation of the median through the vertex R.
Answer:
Question 55:
Find the equation of the line through (–3, 5) and perpendicular to the line through the points (2, 5) and (–3, 6).
Answer:
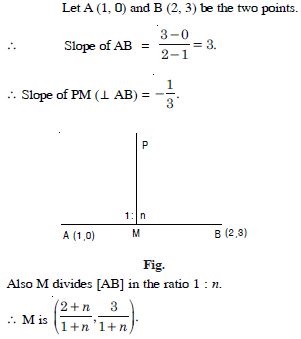
Question 56:
A line perpendicular to the line segment joining the points (1, 0) and (2, 3) divides it in the ratio 1 : n. Find the equation of the line.
Answer:
Question 57:
Find the equation of a line that cuts off equal intercepts on the co-ordinate axes and passes through the point (2, 3).
Answer:
Question 58:
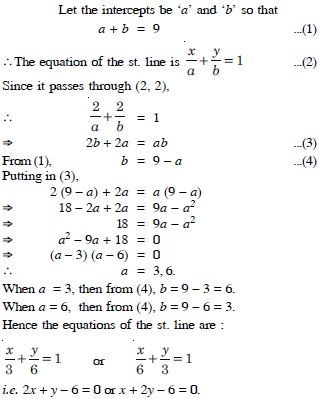
Find the equation of a straight line passing through the point (2, 2) such that the sum of its intercepts on the axes is 9.
Answer:
Question 59:
Answer:
Question 60:
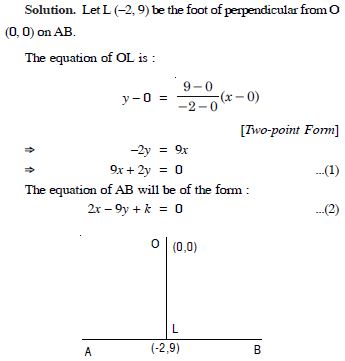
The perpendicular from the origin to a line meets it at the point (–2, 9), find the equation of the line.
Answer:
Question 61:
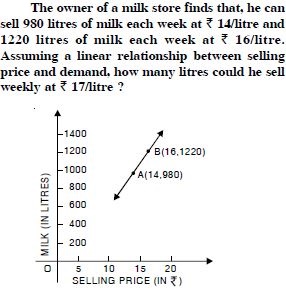
The length L (in centimetres) of a copper rod is a linear function of its Celsius temperature C. In an experiment, if L = 124·942 when C = 20 and L = 125·134 when C = 110, express L in terms of C.
Answer:
Assuming celsius (C) along the x-axis and length
L along the y-axis, we have the relation :
L = m C + k ...(1) [Form : y = mx + c]
When C = 20, L = 124·942
124·942 = m (20) + k ...(2)
When C = 110, L = 125·134
125·134 = m (110) + k ...(3)
Subtracting (2) from (3),
0·192 = 90 m
Putting in (3),
125·134 = 110 (0·213) + k
k = 125·134 – 23·430
= 101·704.
Putting in (1),
L = 0·213 C + 101·704,
which expresses L in terms of C.
Question 62:
Answer:
Question 63:
Answer:
Question 64:
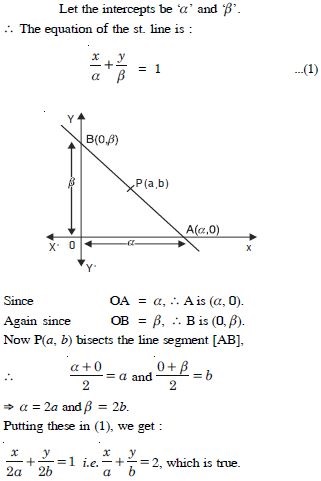
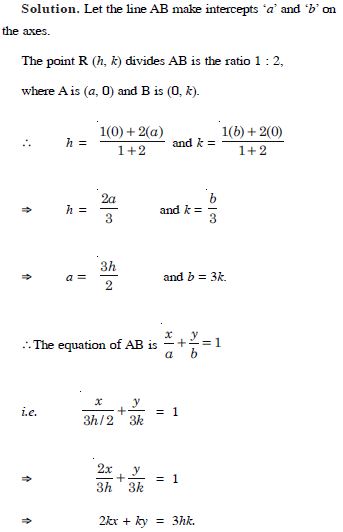
Point R (h, k) divides a line segment between the axes in the ratio 1 : 2. Find the equation of the line.
Answer:
Question 65:
By using the concept of the equation of a line, prove that three points (3, 0), (–2, –2) and (8, 2) are collinear.
Answer:
Question 66:
Reduce the following equations into slope-intercept
form and find their slopes and the y-intercepts :
x + 7y = 0
Answer:
Question 67:
Reduce the following equations into slope-intercept
form and find their slopes and the y-intercepts :
6x + 3y – 5 = 0
Answer:
Question 68:
Reduce the following equations into slope-intercept
form and find their slopes and the y-intercepts :
y = 0
Answer:
The given equation is y = 0
y = 0.x + 0 [Form : y = mx + c]
Slope = 0 and y-intercept = 0.
Question 69:
Reduce the following equations into intercept form and
find their intercepts on the axes :
3x + 2y – 12 = 0
Answer:
Question 70:
Reduce the following equations into intercept form and
find their intercepts on the axes :
4x – 3y = 6
Answer:
Question 71:
Reduce the following equations into intercept form and
find their intercepts on the axes :
3y + 2 = 0
Answer:
Question 72:
Reduce the following equations into normal form. Find their perpendicular distance from the origin and angle between perpendicular and the positive x-axis.
Answer:
Question 73:
Reduce the following equations into normal form. Find
their perpendicular distance from the origin and angle
between perpendicular and the positive x-axis.
y – 2 = 0
Answer:
Question 74:
Reduce the following equations into normal form. Find
their perpendicular distance from the origin and angle
between perpendicular and the positive x-axis.
x – y = 4
Answer:
Question 75:
Find the distance of the point (– 1, 1) from the
line :
12 (x + 6) = 5 (y – 2).
Answer:
The given line is :
12 (x + 6) = 5 (y – 2)
12x + 72 = 5y – 10
12x – 5y + 82 = 0 ....(1)
Distance of (– 1, 1) from line (1)
Question 76:
Answer:
Question 77:
Find the distance between the parallel lines :
15x + 8y – 34 = 0 and 15x + 8y + 31 = 0
Answer:
Question 78:
Find the distance between the parallel lines :
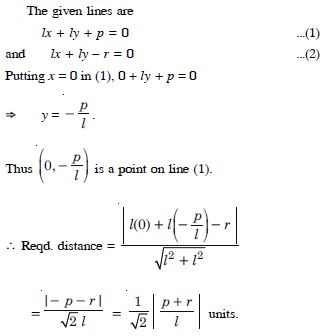
l (x + y) + p = 0 and l (x + y) – r = 0
Answer:
Question 79:
Find the equation of the line parallel to the line 3x – 4y + 2 = 0 and passing through the point (– 2, 3).
Answer:
Any line parallel to 3x – 4y + 2 = 0 ...(1)
is 3x – 4y + k = 0 ...(2)
Since (2) passes thro’ (– 2, 3),
3 (– 2) – 4 (3) + k = 0 k = 6 + 12 = 18.
Putting in (2), 3x – 4y + 18 = 0,
which is the reqd. equation.
Question 80:
Find equation of the line perpendicular to the line x – 7y + 5 = 0 and having x-intercept 3.
Answer:
The given line is x – 7y + 5 = 0 ...(1)
Since the x-intercept is 3,
the reqd. line passes thro’ (3, 0).
Any line perpendicular to (1) is :
7x + y + k = 0 ...(2)
Since (2) passes thro’ (3, 0),
7 (3) + 0 + k = 0 k = – 21.
Putting is (2), 7x + y – 21 = 0,
which is the reqd. equation.
Question 81:
Answer:
Question 82:
The line through the points (h, 3) and (4, 1) intersects the line 7x – 9y – 19 = 0 at right angle. Find the value of h.
Answer:
Question 83:
Answer:
Question 84:
Two lines passing through the point (2, 3) intersect each other at an angle of 60°. If the slope of one line is 2, find the equation of the other line
Answer:
Question 85:
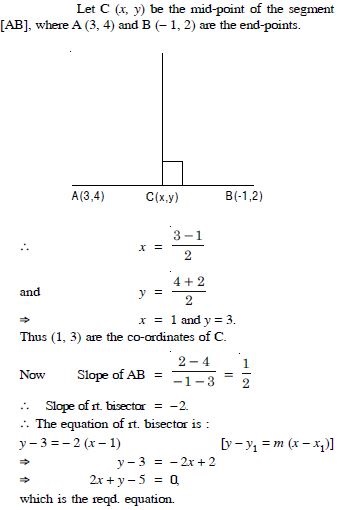
Find the equation of right-bisector of segment joining (3, 4) and (– 1, 2).
Answer:
Question 86:
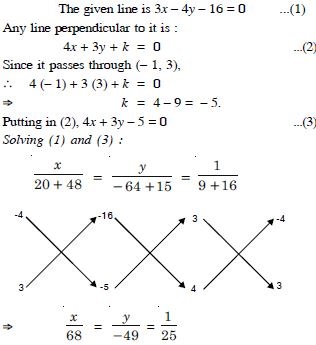
Find the co-ordinates of the foot of perpendicular from a point (– 1, 3) to the line 3x – 4y – 16 = 0.
Answer:
Question 87:
The perpendicular from the origin to the line y = mx + c meets it at the point (– 1, 2). Find the values of m and c.
Answer:
Question 88:
Answer:
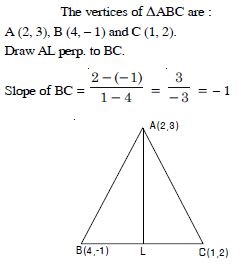
Question 89:
In the triangle ABC with vertices A (2, 3), B (4, – 1) and C (1, 2), find the equation and length of altitude from the vertex A.