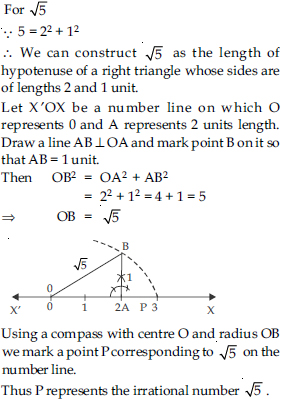
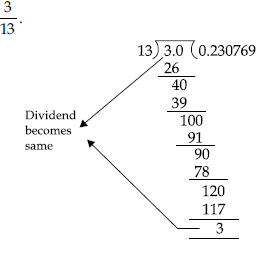
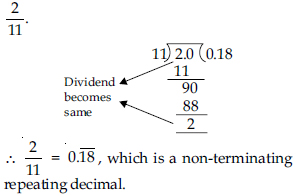
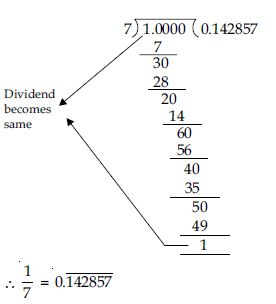
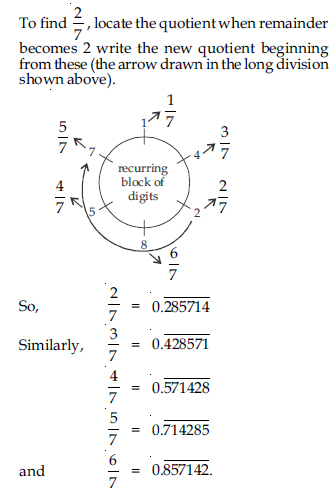
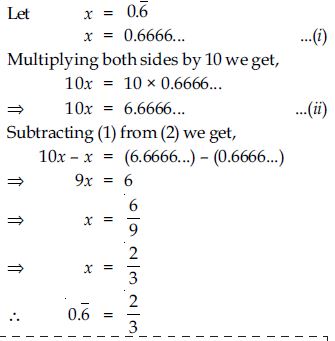
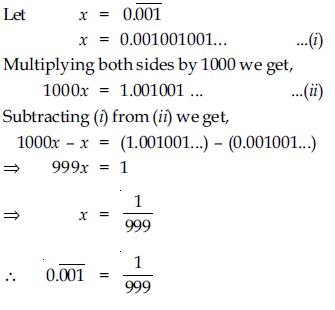
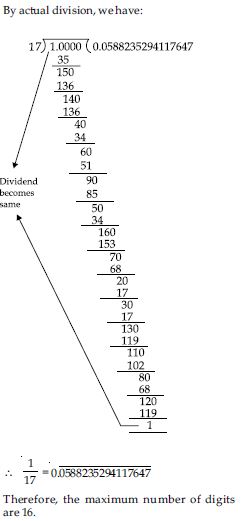
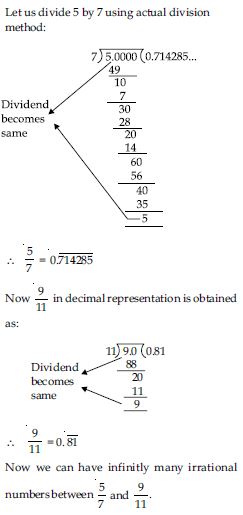
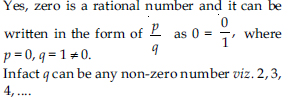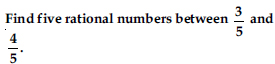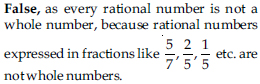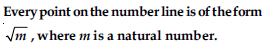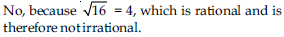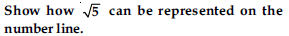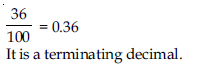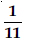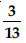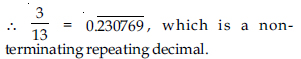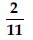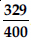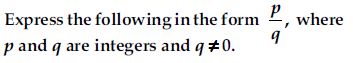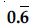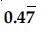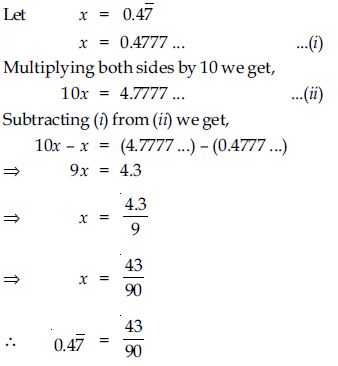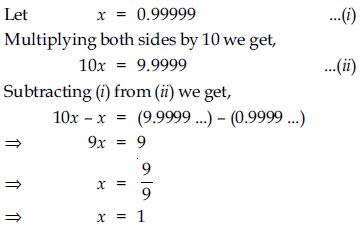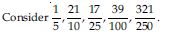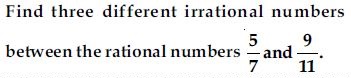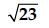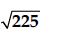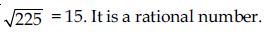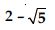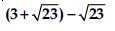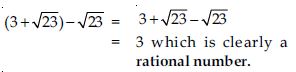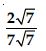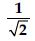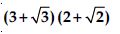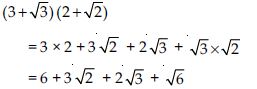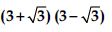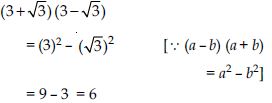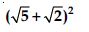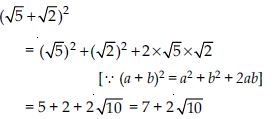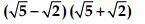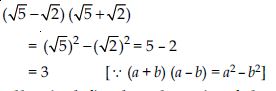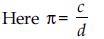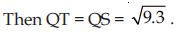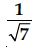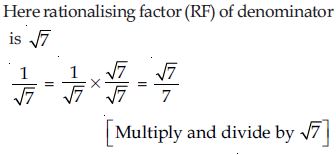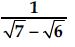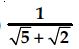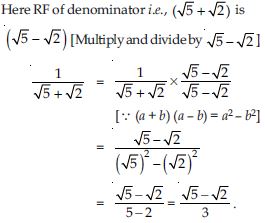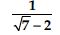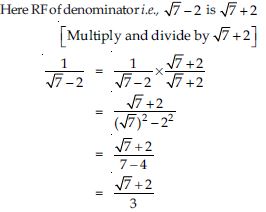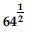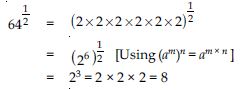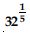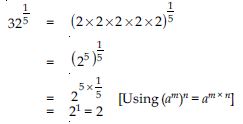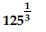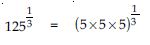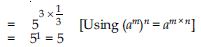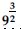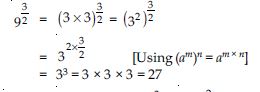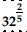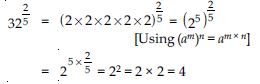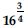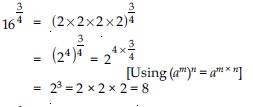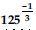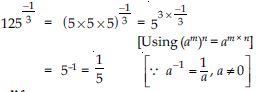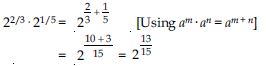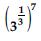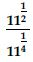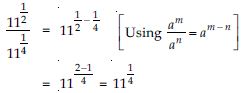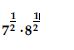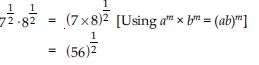Question 1:
Is zero a rational number? Can you write it in the form p/q, where p and q are integers and q 0?
Answer:
Question 2:
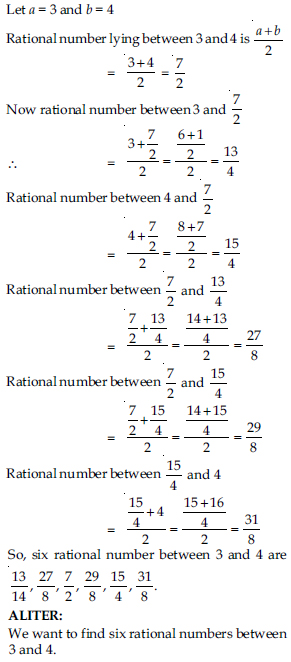
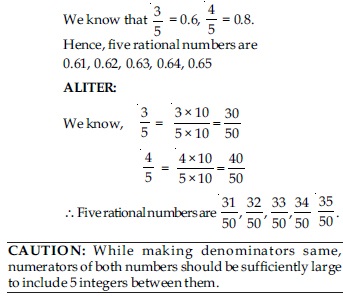
Find six rational numbers between 3 and 4.
Answer:
Question 3:
Answer:
Question 4:
Every natural number is a whole number.
TRUE
FALSE
Answer:
True, since, collection of whole numbers contains all natural numbers.
Question 5:
Every integer is a whole number.
TRUE
FALSE
Answer:
False, as every integer is not a whole number, because negative integers are not whole numbers.
Question 6:
Every rational number is a whole number.
TRUE
FALSE
Answer:
Question 7:
Every irrational number is a real number.
TRUE
FALSE
Answer:
True.
Justification: Because set of rational and irrational numbers constitute the
set of real numbers. In other words all irrational numbers are real numbers. Hence, the
statement ‘every irrational number is a real number’ is true.
Question 8:
Answer:
False.
Justification: – 3, – 5, – 9 are all real numbers but none of these is square
root of any natural number.
Question 9:
Every real number is an irrational number.
TRUE
FALSE
Answer:
False.
Justification: 5, 7, 8, 11 are all real numbers but none is irrational.
Question 10:
Are the square roots of all positive integers irrational? If not, give an example of the square root of a number that is a rational number.
Answer:
Question 11:
Answer:
Question 12:
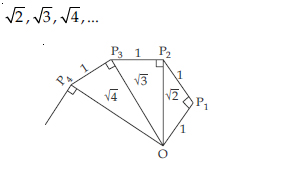
Classroom activity (Constructing the ‘square root spiral’):
Answer:
Take a large sheet of paper and construct the ‘square root spiral’ in the following fashion. Start with a point O and draw a line segment OP1 of unit length. Draw a line segment P1P2 perpendicular to OP1 of unit length [see figure]. Now draw a line segment P2P3 perpendicular to OP2. Then draw a line segment P3P4 perpendicular to OP3. Continuing in this manner, you can get the line segment Pn–1Pn by drawing a line segment of unit length perpendicular to OP n–1 . In this manner, you will have created the points P2, P3, ..., Pn, ... and joined them to create a beautiful spiral depicting
Question 13:
Write the following in decimal form and say what kind of decimal expansion each has:
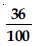
Answer:
Question 14:
Write the following in decimal form and say what kind of decimal expansion each has:
Answer:
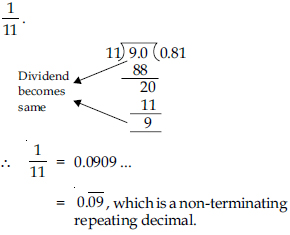
Question 15:
Write the following in decimal form and say what kind of decimal expansion each has:
Answer:
Question 16:
Write the following in decimal form and say what kind of decimal expansion each has:
Answer:
Question 17:
Write the following in decimal form and say what kind of decimal expansion each has:
Answer:
Question 18:
Write the following in decimal form and say what kind of decimal expansion each has:
Answer:
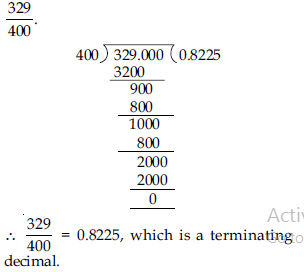
Question 19:

Answer:
Question 20:
Answer:
Note: (i) First shift all non-repeating digits (say m digits in total) LHS by multiplying
both sides with 10m.
(ii) Then shift block of recurring digits (say a block of n recurring digits to LHS by
multiplying both sides with 10n.
Question 21:
Answer:
Question 22:
Answer:
Question 23:
Answer:
Yes, at a glance we are surprised at our answer. But the answer makes sense when we observe that 0.9999... goes on forever. So there is no gap between 1 and 0.9999... and hence they are equal.
Question 24:
Answer:
Question 25:
Answer:
Observe that the denominator (q) of each of above number has prime 2 or 5 or both or their
certain whole number power (index) as its factor.
So, we can say that denominator q of terminating decimal number has prime factors of the
form 2m × 5n where m and n are any whole numbers.
Question 26:
Write three numbers whose decimal expansions are non-terminating non-recurring.
Answer:
Three non-terminating, non-recurring numbers are:
0.201001000100001 ...
0.3516511651116511116...
0.4610792158921821689351...
Note: Answers to this question is not unique. Different answers are possible.
Question 27:
Answer:
Any three of these are:
0.75075007500075000075...,
0.767076700767000... and
0.80800800080000…
Question 28:
Classify the following numbers as rational or irrational:
Answer:
Question 29:
Classify the following numbers as rational or irrational:
Answer:
Question 30:
Classify the following numbers as rational or irrational:
0.3796
Answer:
0.3796. It is a terminating decimal, so it is a rational number.
Question 31:
Classify the following numbers as rational or irrational:
7.478478....
Answer:
Question 32:
Classify the following numbers as rational or irrational:
1.101001000100001...
Answer:
1.101001000100001...
It is a non-terminating non-recurring decimal, so it is an irrational number.
Question 33:
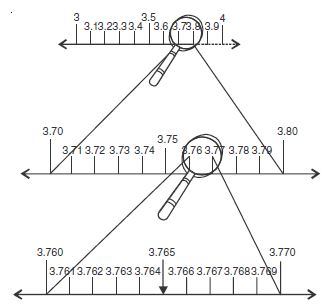
Visualise 3.765 on the number line, using successive magnification.
Answer:
Step (i) The given number lies between 3 and 4.
Step (ii) Magnify the interval between 3 and 4 divided is into 10 equal parts.
Step (iii) The given number lies between 3.7 and 3.8.
Step (iv) Divide the interval 3.7 and 3.8 into ten equal parts and magnify it.
Step (v) The given number lies between 3.76 and 3.77.
Step (vi) Magnify the interval between 3.76 and 3.77 and divide it into ten equal parts.
Step (vii) 3.765 is the fifth division in the magnification.
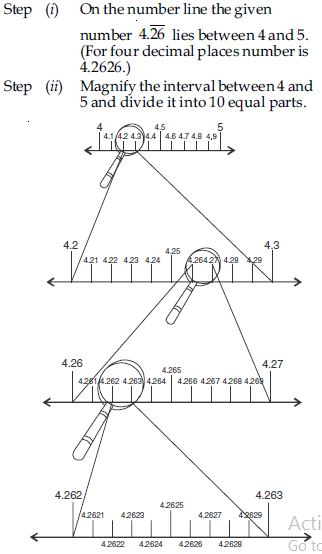
Question 34:
Answer:
Step (iii) The given number 4.2626 lies between 4.2 and 4.3.
Step (iv) Magnify the interval between 4.2 and 4.3 and divide it into ten equal parts.
Step (v) The given number falls between 4.26 and 4.27.
Step (vi) Magnify the interval between 4.26 and 4.27 and divide it into ten equal parts.
Step (vii) The given number lies between 4.262 and 4.263.
Step (viii) Magnify the interval between 4.262 and 4.263 and divide it into ten equal parts.
Question 35:
Classify the following numbers as rational or irrational :
Answer:
Question 36:
Classify the following numbers as rational or irrational :
Answer:
Question 37:
Classify the following numbers as rational or irrational :
Answer:
Question 38:
Classify the following numbers as rational or irrational :
Answer:
Question 39:
Classify the following numbers as rational or irrational :
2
Answer:
In given number; 2 is a non-zero rational number and is an irrational number. As we know that product of a non-zero rational number and
an irrational number is always an irrational number.
So, 2 is an irrational number.
Question 40:
Simplify each of the following expressions:
Answer:
Question 41:
Simplify each of the following expressions:
Answer:
Question 42:
Simplify each of the following expressions:
Answer:
Question 43:
Simplify each of the following expressions:
Answer:
Question 44:
Answer:
As we know that quotient of a non-zero rational number and an irrational number or quotient
of an irrational number with nonzero rational number is always an irrational number.
Therefore, there is no contradiction as either c or d are irrational hence, is an irrational number.
Eye Opener:
The value of up to 20 decimal places is 3.14159 26535 89893 23846... The value of has been calculated up to 100265 digits, but no sign of recurrence of digits was
found. It is thus not a recurring decimal number. So is an irrational number.
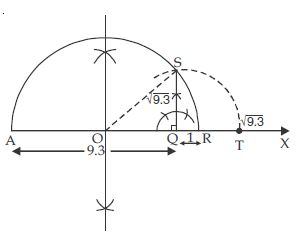
Question 45:
Answer:
(i) Draw a ray AX.
(ii) Mark a point Q on the ray such that AQ = 9.3 units.
(iii) From Q, mark a point R such that QR = 1 unit.
(iv) Draw perpendicular bisector of AR to obtain mid-point. Let us mark it as O.
(v) Taking O as centre and OR as radius draw a semicircle.
(vi) Draw QS ⊥ AX which cuts semicircle at S.
(vii) Taking Q as centre and QS as radius draw an arc cutting AX at T.
Question 46:
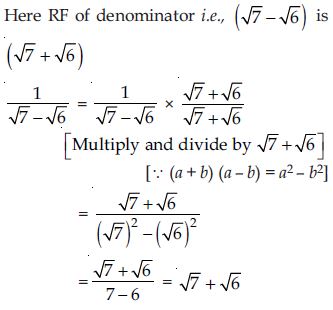
Rationalise the denominators of the following:
Answer:
Question 47:
Rationalise the denominators of the following:
Answer:
Question 48:
Rationalise the denominators of the following:
Answer:
Question 49:
Rationalise the denominators of the following:
Answer:
Question 50:
Find:
Answer:
Question 51:
Find:
Answer:
Question 52:
Find:
Answer:
Question 53:
Find:
Answer:
Question 54:
Find:
Answer:
Question 55:
Find:
Answer:
Question 56:
Find:
Answer:
Question 57:
Simplify :
22/3· 21/5
Answer:
Question 58:
Simplify :
Answer:
Question 59:
Simplify :
Answer:
Question 60:
Simplify :